Droplets are fundamental to fluid transport on solids. Typical examples include 3D printing with relevance to rapid prototyping, self-cleansing surfaces for enhanced solar cell efficiency, microfluidics, spray cooling for high heat flux applications, drop atomization for drug delivery (aerosol) methods, and energy harvesting technologies, all of which involve the motion of droplets on scales where surface tension dominates.
Constrained drops
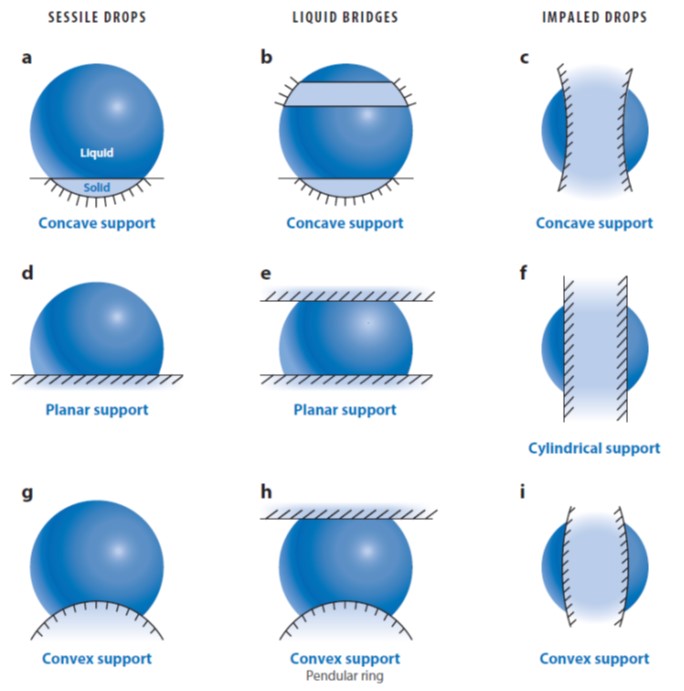
A free drop will oscillate with characteristic frequency and mode shape predicted by Lord Rayleigh (1879). These predictions have been verified experimentally in both terrestrial and low-gravity situations. When the drop is constrained through contact with a solid surface, the frequency spectrum shifts and can `activate’ previously inaccessible low-frequency modes corresponding to motion of the drop’s center-of-mass, through a symmetry breaking mechanism. This can formalized mathematically via boundary homotopy into liquid bridges and impaled drops, as shown in the Figure on the right. Here the spectrum depends upon the dynamic wetting conditions through the static contact-angle and mobility of the three-phase contact-line.
Sessile drops
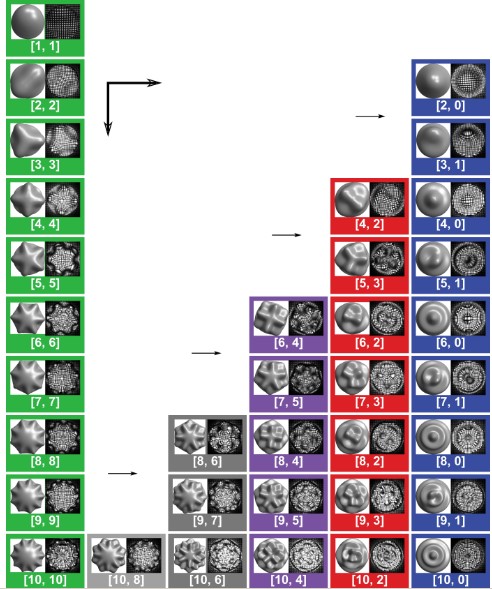
The sessile drop is the canonical problem upon which many modern fluid transport applications are based. For the sessile drop, the spectrum depends upon both the wetting properties of the solid substrate and the mobility of the three-phase contact-line (spreading). Modal shapes can be distinguished by a polar k and azimuthal l wavenumber pair (k,l). In experiment, the sequence of modes observed during a frequency sweep can become distorted; i.e. the (8,8) mode has a lower resonance frequency than the (7,3) mode. This stands in contrast to the free drop (Rayleigh 1879), which preserves spectral ordering. The distorted spectrum can be understood by recasting the system in a symmetry-breaking framework organized by a `periodic table of droplet motions’. This classification is profound in implication and refreshing in originality.
Among other motions, we have discovered a new fluid instability deemed the ‘walking instability’ that is fundamental to fluid transport on solids. Here, under certain circumstances, the potential energy stored in the liquid shape can be converted into fluid motion (kinetic energy).
Ultrasonically-levitated drops
Free drop oscillations can be realized under terrestrial conditions through ultrasonic levitation (cf. Figure (right)). Material properties can be inferred from the frequency response of an amplitude-modulated drop in the so-called `drop vibration rheometer’. For a Newtonian fluid, the resonance frequency is related to the surface tension and the bandwidth to the fluid viscosity. The soft agarose gels used in many bioprinting applications are distinguished in that their resistance to motion is due to both surface tension and elasticity. By tuning the elasticity, it is possible to observe the transition from capillary-dominated to elasticity-dominated motions for these elastocapillary drop oscillations. This noteworthy technique can be used to determine the surface tension of soft gels, a situation where the classical techniques, such as the Wilhelmy plate or pendant drop methods, fail. For complex fluids with a frequency-dependent rheology, the method becomes more complicated and new models are needed.
Leidenfrost drops
Leidenfrost drops can levitate on a vapor layer. When that drop is constrained to lie at the bottom of a heated bowl, spontaneous oscillations of the free surface occur (cf. Figure below). The dynamics can be complex and include mode doubling phenomena.